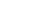Simplify Square Root of 1050

Here we will show you step-by-step how to simplify the square root of 1050. The square root of 1050 can be written as follows:


	√	1050

The √ symbol is called the radical sign. To simplify the square root of 1050 means to get the simplest radical form of √1050.

Step 1: List Factors
List the factors of 1050 like so:

1, 2, 3, 5, 6, 7, 10, 14, 15, 21, 25, 30, 35, 42, 50, 70, 75, 105, 150, 175, 210, 350, 525, 1050

Step 2: Find Perfect Squares
Identify the perfect squares* from the list of factors above:

1, 25

Step 3: Divide
Divide 1050 by the largest perfect square you found in the previous step:

1050 / 25 = 42

Step 4: Calculate
Calculate the square root of the largest perfect square:

√25 = 5

Step 5: Get Answer
Put Steps 3 and 4 together to get the square root of 1050 in its simplest form:


5	√	42

Simplify Square Root Calculator
Please enter another Square Root for us to simplify:

Decimal Form
Square Root of 1050 in Decimal form rounded to nearest 5 decimals:

32.4037

Exponent Form
Square Root of 1050 written with Exponent instead of Radical:

1050^½ = 5 x 42^½

Simplify Square Root of 1051
The answer to Simplify Square Root of 1050 is not the only problem we solved. Go here for the next problem on our list.

* List of Perfect Squares